확률론 S01.8 Countable and Uncountable Sets, S01.9 Proof That a Set of Real Numbers is Uncountable

집합 관련 내용은 아마 다른 친구들은 집합론 시간에 배웠을 것이다

- 대충 countable set, uncountable set은 들어봤는데, 내가 좀 더 자세하게 들은것은 이 강의에서다.

 

https://www.youtube.com/watch?v=MqocbJ-FPo0&list=PLUl4u3cNGP60hI9ATjSFgLZpbNJ7myAg6&index=19 

 

 

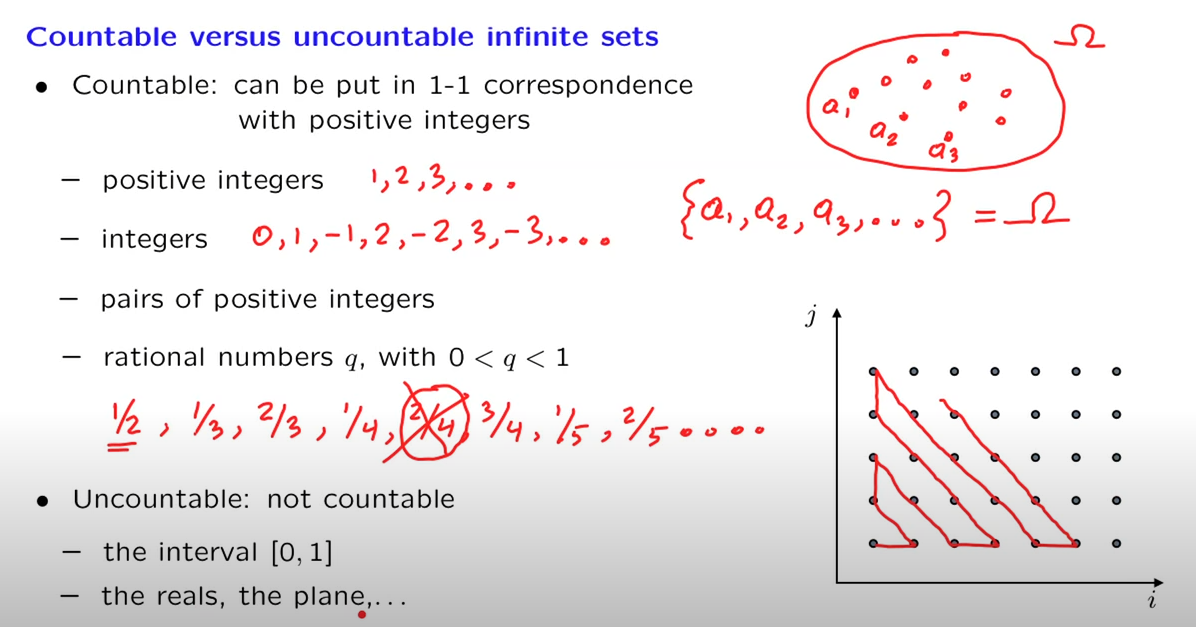
Countable set :

자연수와 1대1 매칭이 되는 집합을 countable set이라고 "정의"

positive integers, integers, pair of positive integers, 0<q<1인 유리수 q

- "0<q<1인 유리수 q"는 pair of positive integers에 해당된다.

 

Uncountable set : the interval [0,1], the reals, the plane ...

 

https://www.youtube.com/watch?v=YIZd23zGV3M&list=PLUl4u3cNGP60hI9ATjSFgLZpbNJ7myAg6&index=20 

 

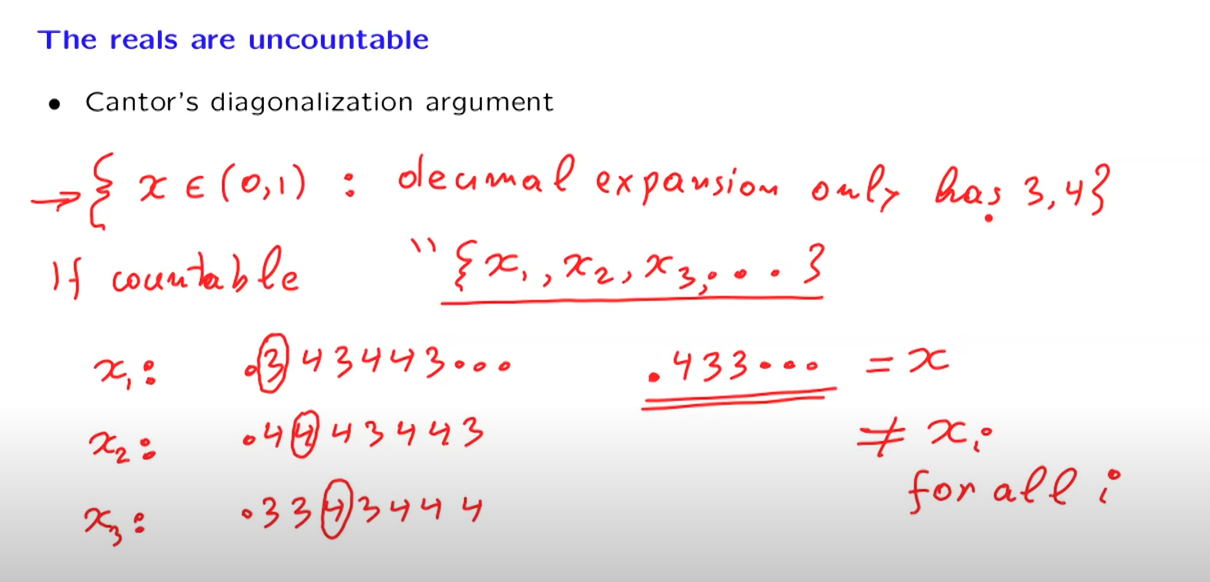
실수집합이 uncountable infinity set인것을 가볍게 증명해준다.

 

countable set: 자연수에 1대1 matching이 되는 집합.

 

proof:

실수집합을 countable이라고 가정하자.

0<x<1, x는 3,4만 가지는 수라고 하자

 

그러면

x1: 0.343443...

x2: 0.4443443....

x3: 0.3343444...

 

어떤 수를 잡아도 그 수사이의 수를 또 발견할 수 있다.

 

따라서 실수집합은 자연수에 1:1 matching을 할 수 없다.

-> 따라서 실수집합은 uncountable이다.

 

[추가자료]

자연수 집합의 크기와 정수 집합의 크기는 같다.

https://blog.naver.com/duxogn08/221154831584

[수학][미적분] 자연수의 개수와 정수의 개수는 같다?

자연수 집합의 크기와 유리수 집합의 크기는 같다.

https://blog.naver.com/duxogn08/221156225081

[수학][미적분] 자연수의 개수와 유리수의 개수는 같다? (무한집합의 크기 비교)